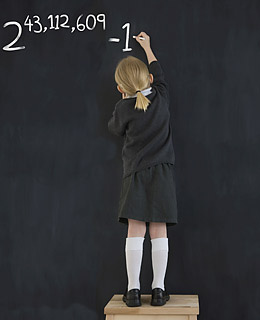On January 25th 2013, in Orlando, Florida, a computer running as part of the Great Internet Mersenne Prime Search (GIMPS) discovered the latest, largest known prime number.

They are called Mersenne primes after Marin Mersenne (1588-1648), a French monk. He acted as a communication hub for mathematicians and scientists of the day, sharing ideas between the likes of Descartes, Fermat, Pascal, Huygens and Galileo. Born to a working class family he went to the same school as Descartes. Eventually he joined “The Order of Minims”, who considered themselves the least (minimi) of all religions on earth and lived a very simple life.
One of his works was “L’harmonie universelle”, where he was the first to publish the laws relating to the vibrating string: its frequency being proportional to the square root of the tension, and inversely proportional to the length.
At the time, many mathematicians were obsessed with finding a pattern in prime numbers. Marcus du Sautoy, in “The Music of the Primes”, suggests that his interest in music may have given him insight into the formula for Mersenne primes: 2n-1. If you double the frequency of a note, you go up an octave, creating harmonic notes. A shift of 1 might be expected to create a very dissonant note, not compatible with any previous frequency – a ‘prime’ note. However, the formula could also have been a result of the search for perfect numbers. A perfect number, such as 28, is the sum of its factors other than itself (1,2,4,7,14).
It was Euclid that showed that whenever the sum of powers of 2 is a prime number, then you can create a perfect number by multiplying the sum by the highest double added. Since the sum of powers of two is 2n – 1 (sum of a Geometric Series), in modern notation, Euclid showed that:
Whenever 2n – 1 is prime, then (2n – 1) x 2n-1 is perfect.
For example, 1 + 2 + 4 = 7 is prime, so 7 x 4 = 28 is perfect. However, 1 + 2 + 4 + 8 = 15 is not prime, so no perfect number can be generated. The next sum works (31) and this generates a perfect number (31 x 16 = 496).
So, the hunt for perfect numbers – which were felt to have religious significance – became a search for when 2n – 1 was prime. In 1644, Mersenne conjectured that this was the case when n = 2, 3, 5, 7, 13, 19, 31, 67, 127 and 257. It was quite a feat, at the time, to have found that 2047 (211-1) is not prime as 2047 = 23 x 89. No one knows how Mersenne came up with the list and it was only in 1876 that Edouard Lucas devised a method for checking Mersenne numbers.
He found 267-1 was not prime but 261-1 was. Some have suggested this was a misprint in the original publication! It was also found that some numbers not on the list do produce primes (89 and 107). It was found that n=127 works and this remained the largest Mersenne prime until computers were invented. Only in 1952 was it found that 257 did not work. Whilst the Lucas method can show whether a Mersenne number is a prime or not, it doesn’t show how non-primes can be factorised. In 1903, Frank Cole gave a talk at the American Mathematical Society. Without saying a word he wrote:
267 – 1 = 193,707,721 x 761,838,257,287.
He got a standing ovation.
It is very difficult to factorise large numbers with large prime factors. This is why the hunt for ever bigger prime numbers is important. Prime numbers are used in on-line purchases. Credit card numbers are encoded by using numbers that can only be factorised into two primes of, at least, 60 digits each.
As e-commerce has grown, so has the need for bigger primes. The latest 257,885,161-1 has 17,425,170 digits.There is prize money available for large primes and the Electronic Frontier Foundation (www.eff.org) is now offering $150,000 for the first prime over 100 million digits and $250,000 for one over a billion digits. You can help the search by downloading the GIMPS software (www.mersenne.org). They will award you $3,000 for a new prime with less than 100 million digits. Happy prime hunting!
Don Hoyle
Mathematics Matters